Use of the Schulz or Gamma distribution in the description of particle sizes facilitates calculation of analytic polydisperse form factors using Laplace transforms, 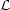 [f(u)]. Here, the Laplace transform approach is combined with the separated form factor (SFF) approximation [Kiselev et al. (2002). Appl. Phys. A, 74, S1654-S1656] to obtain expressions for form factors, P(q), for polydisperse spherical vesicles with various forms of membrane scattering length density (SLD) profile. The SFF approximation is tested against exact form factors that have been numerically integrated over the size distribution, and is shown to represent the vesicle form factor accurately for typical vesicle sizes and membrane thicknesses. Finally, various model SLD profiles are used with the SFF approximation to fit experimental small-angle neutron scattering (SANS) curves from extruded unilamellar vesicles.
[f(u)]. Here, the Laplace transform approach is combined with the separated form factor (SFF) approximation [Kiselev et al. (2002). Appl. Phys. A, 74, S1654-S1656] to obtain expressions for form factors, P(q), for polydisperse spherical vesicles with various forms of membrane scattering length density (SLD) profile. The SFF approximation is tested against exact form factors that have been numerically integrated over the size distribution, and is shown to represent the vesicle form factor accurately for typical vesicle sizes and membrane thicknesses. Finally, various model SLD profiles are used with the SFF approximation to fit experimental small-angle neutron scattering (SANS) curves from extruded unilamellar vesicles.

 journal menu
journal menu